BALANCING OF ROTATING MASSES
When a mass moves along a circular path, it experiences a centripetal acceleration and a force is required to produce it. An equal and opposite force called centrifugal force acts radially outwards and is a disturbing force on the axis of rotation. The magnitude of this
remains constant but the direction changes with the rotation of the mass.
In a revolving rotor, the centrifugal force remains balanced as long as the centre of the mass of rotor lies on the axis of rotation of the shaft. When this does not happen, there is an eccentricity and an unbalance force is produced. This type of unbalance is common in steam turbine rotors, engine crankshafts, rotors of compressors, centrifugal pumps etc.
When a mass moves along a circular path, it experiences a centripetal acceleration and a force is required to produce it. An equal and opposite force called centrifugal force acts radially outwards and is a disturbing force on the axis of rotation. The magnitude of this
remains constant but the direction changes with the rotation of the mass.
In a revolving rotor, the centrifugal force remains balanced as long as the centre of the mass of rotor lies on the axis of rotation of the shaft. When this does not happen, there is an eccentricity and an unbalance force is produced. This type of unbalance is common in steam turbine rotors, engine crankshafts, rotors of compressors, centrifugal pumps etc.
BALANCING OF A SINGLE ROTATING
MASS ROTATING IN THE SAME PLANE
Consider a disturbing mass m1 which is attached to a shaft rotating at ω rad/s.
Let
r1 :- radius of rotation of the mass m1
distance between the axis of rotation of the shaft and the centre of gravity of the mass m1
The centrifugal force exerted by mass m1 on the shaft is given by,
The centrifugal force exerted by mass m1 on the shaft is given by,
This force acts radially outwards and produces bending moment on the shaft. In order to
counteract the effect of this force Fc1 , a balancing mass m2 may be attached in the same
plane of rotation of the disturbing mass m1 such that the centrifugal forces due to the two
masses are equal and opposite.
MASS ROTATING IN THE SAME PLANE
Consider a disturbing mass m1 which is attached to a shaft rotating at ω rad/s.
Let
r1 :- radius of rotation of the mass m1
distance between the axis of rotation of the shaft and the centre of gravity of the mass m1
The centrifugal force exerted by mass m1 on the shaft is given by,
The centrifugal force exerted by mass m1 on the shaft is given by,
masses are equal and opposite.
Let,
r2:- radius of rotationof the mass m2
distance between the axis of rotationof the shaft and
the centre of gravity of the mass m2
Therefore the centrifugal force due to mass m2 will be,
The product m2r2
can be split up in any convenient way. As for as possible the radius
of rotation of mass m2 that is r2 is generally made large in order to reduce the balancing
mass m2.
r2:- radius of rotationof the mass m2
distance between the axis of rotationof the shaft and
the centre of gravity of the mass m2
Therefore the centrifugal force due to mass m2 will be,
BALANCING OF A SINGLE ROTATING MASS BY TWO MASSES ROTATING
IN DIFFERENT PLANES.
There are two possibilities while attaching two balancing masses:
1. The plane of the disturbing mass may be in between the planes of the two
balancing masses.
2. The plane of the disturbing mass may be on the left or right side of two planes
containing the balancing masses.
In order to balance a single rotating mass by two masses rotating in different planes which are parallel to the plane of rotation of the disturbing mass i) the net dynamic force acting on the shaft must be equal to zero, i.e. the centre of the masses of the system must lie on the axis of rotation and this is the condition for static balancing ii) the net couple due to the dynamic forces acting on the shaft must be equal to zero, i.e. the algebraic sum of the moments about any point in the plane must be zero.
The conditions i) and ii) together give dynamic balancing.
IN DIFFERENT PLANES.
There are two possibilities while attaching two balancing masses:
1. The plane of the disturbing mass may be in between the planes of the two
balancing masses.
2. The plane of the disturbing mass may be on the left or right side of two planes
containing the balancing masses.
In order to balance a single rotating mass by two masses rotating in different planes which are parallel to the plane of rotation of the disturbing mass i) the net dynamic force acting on the shaft must be equal to zero, i.e. the centre of the masses of the system must lie on the axis of rotation and this is the condition for static balancing ii) the net couple due to the dynamic forces acting on the shaft must be equal to zero, i.e. the algebraic sum of the moments about any point in the plane must be zero.
The conditions i) and ii) together give dynamic balancing.
1. THE PLANE OF THE DISTURBING MASS LIES IN BETWEEN THE PLANES
OF THE TWO BALANCING MASSES.
Consider the disturbing mass m lying in a plane A which is to be balanced by two
rotating masses m1 and m2 lying in two different planes M and N which are parallel to
the plane A as shown.
Let r, r1 and r2 be the radii of rotation of the masses in planes A, M and N respectively.
Let L1, L2 and L be the distance between A and M, A and N, and M and N respectively.
Now,
The centrifugal force exerted by the mass m in plane A will be,
Similarly,
The centrifugal force exerted by the mass m1 in plane M will be,
And the centrifugal force exerted by the mass m2 in plane N will be,
For the condition of static balancing,
Therefore,
Now, to determine the magnitude of balancing force in the plane „M‟ or the dynamic
force at the bearing „O‟ of a shaft, take moments about „ P ‟ which is the point of
intersection of the plane N and the axis of rotation.
Therefore,
OF THE TWO BALANCING MASSES.
Consider the disturbing mass m lying in a plane A which is to be balanced by two
rotating masses m1 and m2 lying in two different planes M and N which are parallel to
the plane A as shown.
Let r, r1 and r2 be the radii of rotation of the masses in planes A, M and N respectively.
Let L1, L2 and L be the distance between A and M, A and N, and M and N respectively.
Now,
The centrifugal force exerted by the mass m in plane A will be,
The centrifugal force exerted by the mass m1 in plane M will be,
force at the bearing „O‟ of a shaft, take moments about „ P ‟ which is the point of
intersection of the plane N and the axis of rotation.
Therefore,
For dynamic balancing equations (5) or (6) must be satisfied along with equation (4).
2. WHEN THE PLANE OF THE DISTURBING MASS LIES ON ONE END OF THE
TWO PLANES CONTAINING THE BALANCING MASSES.
For static balancing,g
For dynamic balance the net dynamic force acting on the shaft and the net couple due to dynamic forces acting on the shaft is equal to zero.
To find the balancing force in the plane „M‟ or the dynamic force at the bearing „O‟ of a
shaft, take moments about „P‟. i.e.
TWO PLANES CONTAINING THE BALANCING MASSES.
For static balancing,g
To find the balancing force in the plane „M‟ or the dynamic force at the bearing „O‟ of a
shaft, take moments about „P‟. i.e.
Therefore,
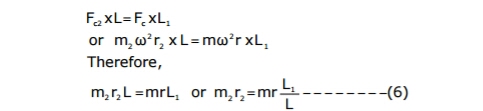
Comments
Post a Comment